Class 12 Chapter 6 (Application Of Derivative) Class Notes part I

APPLICATIONS OF DERIVATIVE

 

SOME IMPORTANT FORMULAE/KEYCONCEPTS

 

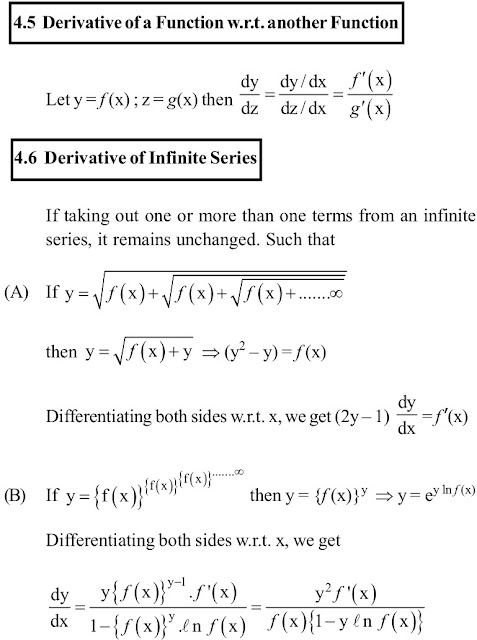
1. RATE OF CHANGE OF QUANTITIES

    

   Whenever one quantity y varies with another quantity x, satisfying some rule y = f(x), then \(\dfrac{{dy}}{{dx}}\) or f'(x) represents the rate of change of y with resprect to x and \({\left[ {\dfrac{{dy}}{{dx}}} \right]_{x = {x_0}}}\) or f'(x₀) represents rate of change of y with resprect to x at x = x₀

    

   EXAMPLE :

   QUESTION :The radius of a circle is increasing at the rate of 0.7 cm/s. What is the rate of increase of its circumference?
   SOLUTION :Let any instant of time t, the radius of circle = r
   Then, circumference C = \(2\pi r\)
   Diff. Both sides w.r.t t, we get

   \(\dfrac{{dC}}{{dt}} = \dfrac{{d(2\pi r)}}{{dt}} = 2\pi \dfrac{{dr}}{{dt}}\)

   \(\dfrac{{dr}}{{dt}} = 0.7\,\,cm/s\) {Given}

   Here, \(\dfrac{{dC}}{{dt}} = 2\pi \times 0.7 = 1.4\pi \,\,\,cm/s\)

2. INCREASING AND DECREASING FUNCTIONS:

   Let I be an open interval contained in the domain of a real valued function f. Then f is said to be

   1. increasing on I, if x₁ < x₂ in I, \( \Rightarrow f({x_1}) \le f({x_2})\) for all \({x_1},{x_2} \in I\)

   2. strictly increasing on I, if x₁ < x₂ in I, \(\Rightarrow f({x_1}) < f({x_2})\,for\,\,all\,{x_1},{x_2} \in I.\)

   3. increasing on I, if x₁ < x₂ in I, \(\Rightarrow f({x_1}) \ge f({x_2})\,for\,\,all\,{x_1},{x_2} \in I.\)

   4. increasing on I, if x₁ < x₂ in I, \(\Rightarrow f({x_1}) > f({x_2})\,for\,\,all\,{x_1},{x_2} \in I.\)

    

   USING THE CONCEPTS OF DERIVATIVES

   1. f is strictly increasing in (a, b) if f ′(x) > 0 for each x \( \in \) (a, b)

   2. f is strictly decreasing in (a, b) if f ′(x) < 0 for each x \( \in \) (a, b)

   3. A function will be increasing (decreasing) in R if it is so in every interval of R.

    

   
   
   

    

   EXAMPLE

   QUESTION : Find the interval in which the function \(f(x) = 2{x^3} - 9{x^2} + 12x + 15\) is:
   (i) strictly increasing (ii) strictly decreasing

   SOLUTION: \(f(x) = 2{x^3} - 9{x^2} + 12x + 15\)

   \(f'(x) = 6({x^2} - 3x + 2)\)

   1. For strictly increasing, f’(x) > 0

      6(x² - 3x + 2) > 0

      (x - 1)(x - 2) > 0

      x< 1 or x > 2

      \(x \in ( - \infty ,1) \cup (2,\infty )\)

      so, f(x) is strictly increasing on \(x \in ( - \infty ,1) \cup (2,\infty )\)

       

   2. for strictly decreasing f’(x) < 0

   6(x² - 3x + 2) < 0

   (x - 1)(x - 2) < 0

   1 < x < 2

   so, f(x) is strictly decreasing on (1, 2).

    

3. TANGENTS AND NORMALS:

   • Slope of the tangent to the curve y = f (x) at the point (x_o, y_o) is given by

     \[{\left[ {\frac{{dy}}{{dx}}} \right]_{({x_0},{y_0})}} = f'({x_0})\]

      

   • The equation of the tangent at (x_o, y_o) to the curve y = f (x) is given by y – y_o = 𝑓′(x_o)(x – x_o).

     
     
     

      

   • Slope of the normal to the curve y = f (x) at (x , y ) = \(\dfrac{1}{{slope\,\,of\,\,tangent\,\,at\,({x_0},{y_0})}}\)

   • Slope of the normal to the curve y = f (x) at (x , y) is given by \(\dfrac{1}{{f'({x_0})}}\)

   • The equation of the normal (x_o, y_o) to the curve y = f (x) is given by \(y - {y_0} = \dfrac{1}{{f'({x_0})}}(x - {x_0})\)

   • If slope of the tangent line is zero, then tan θ = 0 and so θ = 0 which means the tangent line is parallel to the x-axis. In this case, the equation of the tangent at the point (x_o, y_o) is given by y=y_o.

   • If \(\theta \to \dfrac{\pi }{2}\), then \(\tan \theta \to \infty \), which means the tangent line is perpendicular to the y - axis. In this case, the equation of the tangent at (x_o, y_o) is given by x = x_o.

    

   EXAMPLE

   QUESTION: Find the equation of normal to the curve y = x³ + 2x + 6 which are parallel to the line x + 14y + 4 = 0.

   SOLUTION: y = x³ + 2x + 6 

   \(\dfrac{{dy}}{{dx}} = 3{x^2} + 2\)

   Slope of the tangent at (x₁, y₁) = \(3x_1^2 + 2\)

   Slope of normal at point (x₁, y₁) = \(\dfrac{1}{{3x_1^2 + 2}}\)

   Slope of the given line is \(\dfrac{{ - 1}}{{14}}\)

   According to the given condition, Slope of normal = Slope of line

   \(\dfrac{{ - 1}}{{3x_1^2 + 2}} = \dfrac{{ - 1}}{{14}}\)

   \(x = \pm 2\)

   When x₁ = 2, then y₁ = 18 and When x₁ = - 2, then y₁ = - 6

   Equation of normal at (2,18) is: \(y - 18 = \dfrac{{ - 1}}{{14}}(x - 2)\)

   x + 14y = 254 .....(1)

   Equation of normal at (-2, -6) is \(y + 6 = \dfrac{{ - 1}}{{14}}(x + 2)\)

   x + 14y + 86 = 0

    

    

4. Approximations:

   \[f(x + \Delta x) = f(x) + \Delta y\] \[ \,\,\,\,\, \approx f(x) + f'(x) \times \Delta x\,\,\,\,\,\,\,\,\,\,\,\,\,(as\,dx = \Delta x)\]

    

   • Increment \(\Delta y\) in the function y = f(x) corresponding to increment \(\Delta x\) in x is

                \(\Delta y = \dfrac{{dy}}{{dx}}\Delta x\)

   • Relative error in \(y = \dfrac{{\Delta y}}{y}\).

   • Percentage error in \(y = \dfrac{{\Delta y}}{y} \times 100\).

• 
  

  EXAMPLE

  QUESTION: Evaluate \(\sqrt[4]{{81.5}}\)

   

  SOLUTION: \(\sqrt[4]{{81.5}} = \sqrt[4]{{81 + 0.5}}\)

  Let x = 81 and \(\Delta x\) = 0.5

  \[y = \sqrt[4]{x} = \sqrt[4]{{81}}\,\,\,\,\,\,\,\,..........(i)\]

  \[y + \Delta y = \sqrt[4]{{81.5}}\,\,\,\,..........(ii)\]

  \[\Delta y = \sqrt[4]{{81.5}} - \sqrt[4]{x}\]

  \[\sqrt[4]{{81.5}} = \Delta y + \sqrt[4]{x}\]

  using approximation \(\Delta y \approx dy\)

  \[\sqrt[4]{{81.5}} = \frac{{dy}}{{dx}} \times dx + \sqrt[4]{x}\]

  \[ = \frac{1}{4}{x^{\frac{{ - 3}}{4}}} \times 0.5 + 3\]

  \[ = \frac{1}{4} \times {81^{\frac{{ - 3}}{4}}} \times \frac{1}{2} + 3\]

  \[ = \frac{1}{4} \times \frac{1}{{27}} \times \frac{1}{2} + 3 = \frac{1}{{216}} + 3 = 3.0046\]

   

  Click Here for Maxima and Minima
  

   

 

Share:

Read More

Class 12 chapter 5 (Continuity and Differentiability) class notes

♦ Chapter - 5

☞  Continuity and Differentiability 

☑ CONTINUITY 

☑ DEFINITION

⛊ A function f (x) is said to be continuous at x = a; where a ∈ domain of f(x), if

i.e., LHL=RHL = value of a function at x= a

☑  Reasons of discontinuity

⛊ If f (x) is not continuous at x = a, we say that f (x) is discontinuous at x = a.
There are following possibilities of discontinuity

☑ PROPERTIES OF CONTINUOUS FUNCTIONS

☑ THE INTERMEDIATE VALUE THEOREM

⛊ Suppose f(x) is continuous on an interval I, and a and b are any two points of I. Then if y₀ is a number between f(a) and f(b), their exits a number c between a and b such that f(c)=y₀.

☑ Note :-

⛊ That a function f which is continuous in [a, b] possesses the following properties:

( i ) If f (a) and f(b) possess opposite signs, then there exists at least one solution of the equation f(x) =0 in the open interval (a, b).

(ii) If K is any real number between f(a) and f(b), then there exists at least one solution of the equation f (x) = K in the open interval (a, b).

☑  CONTINUITY IN AN INTERVAL

(a) A function f is said to be continuous in (a, b) if f is continuous at each and every point ∈ (a, b).

(b) A function f is said to be continuous in a closed interval [a, b] if:

(1) f is continuous in the open interval (a, b) and

(2) f is right continuous at 'a' i.e. limit ₓ→ₐ⁺ f(x)=f(a) = a finite quantity.

(3) f is left continuous at 'b'; i.e. limit ₓ→b⁻f(x) = f(b) a finite quantity.

☑ A LIST OF CONTINUOUS FUNCTIONS

☑ TYPES OF DISCONTINUITIES



Type-1: (Removable type of discontinuities) 

In case, limit ₓ→c f(x) exists but is not equal to f (c) then the function is said to have a removable discontnuity or discontinuity of the first kind. In this case, we can redefine the function such that limit ₓ→c f(x) = f (c) and make it continuous at x=c. Removable type of discontinuity can be further classified as:

(a) Missing Point Discontinuity :

Where limit ₓ→ₐ f(x) exists finitely but f(a) is not defined.

☑ Isolated Point Discontinuity:

Type-2: (Non-Removable type of discontinuities) 

In case, limit ₓ→ₐ f(x) does not exist, then it is not possible to make the function continuous by redefining it. Such discontinuities are known as non-removable discontinuity or discontinuity of the 2nd kind. Non-removable type of discontinuity can be further classified as :

☑ (a) Finite Discontinuity :

☑ (b) Infinite Discontiunity:

☑ (c) Oscillatory Discontinuity :

From the adjacent graph note that

- f is continuous at x =-1
- f has isolated discontinuity at x = 1
- f has missing point discontinuity at x = 2
- f has non-removable (finite type) discontinity at the origin.

☑ Note :-

⛊ (a) In case of dis-continuity of the second kind the non- negative difference between the value of the RHL at x=a and LHL at x=a is called the jump of discontinuity. A function having a finite number of jumps in a given interval I is called a piece wise continuous or sectionally continuous function in this interval.

(b) All Polynomials, Trigonometrical functions, exponential and Logarithmic functions are continuous in their domains.

(c) If f (x) is continuous and g (x) is discontinuous at x = a then the product function ∅ (x)=f(x). g (x) is not necessarily be discontinuous at x = a. e.g.

(d) If f (x) and g (x) both are discontinuous at x = a then the product function ∅ (x) =f (x). g (x) is not necessarily be discontinuous at x =a. e.g.

☑ DIFFERENTIABILITY 

☑ DEFINITION

⛊ Let f (x) be a real valued function defined on an open interval (a, b) where c ∈ (a, b). Then f(x) is said to be differentiable or derivable at x =c,

☑ DIFFERENTIABILITY IN A SET

1. A function f (x) defined on an open interval (a, b) is said to be differentiable or derivable in open interval (a, b), if it is differentiable at each point of (a, b).

2. A function f (x) defined on closed interval [a, b] is said to be differentiable or derivable. "If f is derivable in the open interval (a, b) and also the end points a and b, then f is said to be derivable in the closed interval [a, b]".

A function f is said to be a differentiable function if it is differentiable at every point of its domain.

☑ Note :-

1. If f (x) and g (x) are derivable at x = a then the functions f(x)+g (x), f(x)-g(x), f(x). g (x) will also be derivable at x=a and if g (a) ≠ 0 then the function f (x)/g(x) will also be derivable at x=a.

2. If f (x) is differentiable at x = a and g (x) is not differentiable at x = a, then the product function F (x) = f (x). g (x) can still be differentiable at x=a.
E.g. f (x) =x and g (x) = |x|.

3. If f (x) and g (x) both are not differentiable at x = a then the product function; F (x) = f(x). g (x) can still be differentiable at x = a.
E.g., f(x) = |x| and g (x) = |x|.

4. If f (x) and g (x) both are not differentiable at x=a then the sum function F (x) =f (x)+g (x) may be a differentiable function.
E.g., f (x) = |x| and g (x)=-|xl.

5. If f(x) is derivable at x= a
    • F' (x) is continuous at x=a.

☑ RELATION B/W CONTINUITY & DIFFERENNINBILINY

In the previous section we have discussed that if a function is differentiable at a point, then it should be continuous at that point and a discontinuous function cannot be differentiable. This fact is proved in the following theorem.

☑ Theorem : If a function is differentiable at a point, it is necessarily continuous at that point. But the converse is not necessarily true,

Or      f(x) is differentiable at x=c
          f(x) is continuous at x = c.

☑ Note. 

⛊ Converse : The converse of the above theorem is not necessarily true i.e., a function may be continuous at a point but may not be differentiable at that point.

E.g., The function f (x) = |x| is continuous at x = 0 but it is not differentiable at x = 0, as shown in the figure.

The figure shows that sharp edge at x = 0 hence, function is not differentiable but continuous at x = 0.

☑ Note:-

 (a) Let f´⁺(a) = p & f´⁻(a)=q where p & q are finite then

(b) If a function f is not differentiable but is continuous at x = a it geometrically implies a sharp corner at X=a.

Theorem 2 : Let fand g be real functions such that fog is defined if g is continuous at x = a and f is continuous at g (a), show that fog is continuous at x = a.

☑ DIFFERENTIATION

☑ DEFINITION

(a) Let us consider a function y=f(x) defined in a certain interval. It has a definite value for each value of the independent variable x in this interval.

Now, the ratio of the increment of the function to the increment in the independent variable,

☑ DERIVATIVE OF STANDARD FUNCTION

☑ THEOREMS ON DERIVATIVES

☑ METHODS OF DIFFERENTIATION

⛊ 4.1 Derivative by using Trigonometrical Substitution

⛊ 4.2 Logarithmic Differentiation

☑ DERIVATIVE OF ORDER TWO & THREE

Let a function y =f (x) be defined on an open interval (a, b). It's derivative, if it exists on (a, b), is a certain function f'(x) [or (dy/dx) or y'] & is called the first derivative of y w.r.t. x. If it happens that the first derivative has a derivative on (a, b) then this derivative is called the second derivative of y w.r.t. x & is denoted by f"(x) or (d²y/dx²) or y".

Similarly, the 3rd order derivative of y w.r.t. x, if it exists, is

Share:

Read More

Class 12 Chapter 4 (determinants) class notes

Chapter - 4

  Determinants 

☞  System of algebraic equations can be expressed in the form of matrices. 

         • Linear Equations Format 

             a1x+b1y=c1

             a2x+b2y=c2

        • Matrix Format:

☞ The values of the variables satisfying all the linear equations in the system, is called solution of system of linear equations. 

☞ If the system of linear equations has a unique solution. This unique solution is called determinant of Solution or det A

☞ Applications of Determinants 

☞ Engineering 

☞ Science 

☞ Economics  

☞ Social Science, etc.

☸ Determinant 

♦ A determinant is defined as a (mapping) unction from the set o square matrices to the set of real numbers 

♦ Every square matrix A is associated with a number, called its determinant 

♦ Denoted by det (A) or |A| or ∆

♦ Only square matrices have determinants.

♦ The matrices which are not square do not have determinants 

♦ For matrix A, |A| is read as determinant of A and not modulus of A. 

☸ Types of Determinant  

♦ 1. First Order Determinant  

  ♦ Let A = [a ] be the matrix of order 1, then determinant of A is defined to be equal to a 

  ♦ If A = [a], then det (A) = |A| = a

♦ 2. Second Order Determinant 

♦ 3. Third Order Determinant 

♦ Can be determined by expressing it in terms of second order determinants

The below method is explained for expansion around Row 1 

The value of the determinant, thus will be the sum of the product of element in line parallel to the diagonal minus the sum of the product of elements in line perpendicular to the line segment. Thus,

The same procedure can be repeated for Row 2, Row 3, Column 1, Column 2, and Column 3 

♦ Note  

   ♦ Expanding a determinant along any row or column gives same value.

   ♦ This method doesn't work for determinants of order greater than 3. 

   ♦ For easier calculations, we shall expand the determinant along that row or column which contains maximum number of zeros 

   ♦ In general, if A = kB where A and B are square matrices of order n, then | A| = kⁿ |B |, where n = 1, 2, 3

☸ Properties of Determinants 

♦ Helps in simplifying its evaluation by obtaining maximum number of zeros in a row or a column. 

♦ These properties are true for determinants of any order. 

☸ Property 1 

♦ The value of the determinant remains unchanged if its rows and columns are interchanged 

♦ Verification:

Expanding ∆₁ along first column, we get 

∆₁ =a₁ (b₂ c₃ - c₂ b₃) - a₂(b₁ c₃ - b₃ c₁) + a₃ (b₁ c₂ - b₂ c₁)

Hence ∆ = ∆₁

♦ Note:

♦ It follows from above property that if A is a square matrix, 

     Then det (A) = det (A'), where A' = transpose of A 

♦ If Ri = ith row and Ci = ith column, then for interchange of row and 

♦ columns, we will symbolically write Ci⇔Ri

☸ Property 2 

♦ If any two rows (or columns) of a determinant are interchanged, then sign of determinant changes. 

♦ Verification :

☸ Property 3 

♦ If any two rows (or columns) of a determinant are identical (all corresponding elements are same), then value of determinant is zero. 

♦ Verification:

♦ If we interchange the identical rows (or columns) of the determinant ∆, then ∆ does not change.

♦ However, by Property 2, it follows that ∆ has changed its sign 

♦ Therefore ∆ = -∆ or ∆ = 0

☸  Property 4 

♦ If each element of a row (or a column) of a determinant is multiplied by a constant k, then its value gets multiplied by k

♦ Verification

☸ Property 5 

♦ If some or all elements of a row or column of a determinant are expressed as sum of two (or more) terms, then the determinant can be expressed as sum of two (or more) determinants.

♦ Verification:

☸ Property 6

♦ If, to each element of any row or column of a determinant, the equimultiples of corresponding elements of other row (or column) are added, then value of determinant remains the same, i.e., the value of determinant remain same if we apply the operation

☸ Property 7 

♦ If each element of a row (or column) of a determinant is zero, then its value is zero

☸ Property 8 

♦ In a determinant, If all the elements on one side of the principal diagonal are Zero's , then the value of the determinant is equal to the product of the elements in the principal diagonal

☸ Area of a Triangle 

♦ Let (x₁,y₁), (X₂, y₂), and (x₃, y₃) be the vertices of a triangle, then

☸ Note

♦ Area is a positive quantity, we always take the absolute value of the determinant .
♦ If area is given, use both positive and negative values of the determinant for caleulation.
♦ The area of the triangle formed by three collinear points is zero.

☸ Minors and Cofactors 

☸ Minor

♦ If the row and column containing the element a₁₁ (i.e., 1st row and 1st column)are removed, we get the second order determinant which is called the Minor of element a₁₁
♦ Minor of an element aij of a determinant is the determinant obtained by deleting its ith row and jth column which element aij lies.

♦ Minor of an element aij is denoted by Mij
♦ Minor of an element of a determinant of order n(n ≥ 2) is a determinant of order n-1

♦ Eg: Find Minor o the element 6 in the determinant A given

☸ Cofactor 

♦ If the minors are multiplied by the proper signs we get cofactors
♦The cofactor of the element aij is Cij = (-1) Mij
♦The signs to be multiplied are given by the rule

♦ Cofactor of 4 is A₁₂ =(-1) M₁₂ =(-1)³(4) =-4

☸ Adjoint and Inverse of a Matrix 

♦ Adjoint of matrix is the transpose of the matrix of cofactors of the given matrix

☸ Theorem 1 

♦ If A be any given square matrix of order n, 

 Then A (adj A) = (adj A) A = A I,

 Where I is the identity matrix of order n 

♦ Verification:

Similarly, we can show (adj A) A = AI
Hence A (adj A) = (adj A) A = AI

☸ Singular & No Singular Matrix:

♦ A square matrix A is said to be singular if |A| = o
♦ A square matrix A is said to be non-singular if |A |≠ 0

☸ Theorem 2 

♦ If A and B are non-singular matrices of the same order, then AB and BA are also non- singular matrices of the same order.

☸ Theorem 3 

♦ The determinant of the product of matrices is equal to product of their respective determinants, that is, AB =|A| |B| , where A and B are square matrices of the same order

☸ Theorem 4 

&dsiams; A square matrix A is invertible if and only if A is non-singular matrix.

♦ Verification

 Let A be invertible matrix of order n and I be the identity matrix of order n. Then, there exists a square matrix B of order n such that AB = BA = I

Now AB = I. So |AB| = I or |A| |B| = 1 (since |I|= 1, |AB|=| A||B|). This gives |A| ≠ 0. Hence A is non-singular.

Conversely, let A be non-singular. Then |A| ≠ 0
Now A (adj A) = (adj A) A = |A| I (Theorem 1)

☸ Applications of Determinants and Matrices 

♦ Used for solving the system of linear equations in two or three variables and for checking the consistency of the system of linear equations.

♦ Consistent system 

♦ A system of equations is said to be consistent if its solution (one or more) exists.

♦ Inconsistent system 

♦ A system of equations is said to be inconsistent if its solution does not exist

☸ Solution of system of linear equations using inverse of a matrix 

♦ Let the system of Equations be as below:

a₁x+b₁y +c₁z=d₁
a₂x +b₂y +c₂z=d₂
a₃x+b₃y+c₃z=d₃

☸ Case I

If A is a non-singular matrix, then its inverse exists.

AX = B
A⁻¹(AX) = A⁻¹B (premultiplying by A⁻¹)
(A⁻¹A)X -A⁻¹B (by associative property)
1X = A⁻¹B
X = A⁻¹B

This matrix equation provides unique solution for the given system of equations as inverse of a matrix is unique. This method of solving system of equations is known as Matrix Method

𘗈  Case II 

If A is a singular matrix, then |A| = 0.
In this case, we calculate (adj A) B.
If (adj A) B ≠ O, (O being zero matrix), then solution does not exist and the system of equations is called inconsistent.
If (adj A) B = O, then system may be either consistent or inconsistent according as the system have either infinitely many solutions or no solution

☸  Summary

For a square matrix A in matrix equation AX = B

♦ |A| ≠ 0, there exists unique solution
♦ |A| = 0 and (adj A) B ≠ 0, then there exists no solution
♦ |A| o and (adj A) B = 0, then system may or may not be consistent.



   

Share:

Read More