Notes of Chapter 10 Circles Class 9th Math
Terms Related to Circles
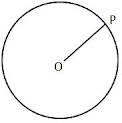
- The locus of a point which moves in a plane in such a manner that its distance from a given fixed point is always constant, is called a circle.
- The fixed point is called the centre and constant distance is called the radius of the circle.
In the figure, ‘O’ is centre and OP = r is a radius. We denote it by C(O, r).
- A line segment, terminating (or having its end points) on the circle is called a chord. A chord, passing through the centre is called a diameter of the circle.
- A line which intersects a circle in two distinct points is called a secant of the circle.
- A line intersecting the circle in exactly one point is called a tangent to the circle.

- In the figure, PQ is a chord, AB is a diameter, XY is a secant and ST is a tangent to the circle at C.
(i) Diameter is the longest chord in a circle.
(ii) Diameter = 2 × Radius
- The length of the complete circle is called its circumference, whereas a piece of a circle between two points is called an arc.
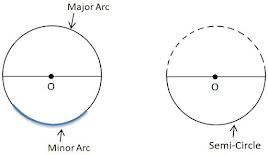
(i) A diameter of a circle divides it into two equal arcs, each of which is called a semicircle.
(ii) If the length of an arc is less than the semicircle, then it is a minor arc, otherwise, it is a major arc.
- The region consisting of all points lying on the circumference of a circle and inside it is called the interior of the circle.
- The region consisting of all points lying outside a circle is called the exterior of the circle.
- The region consisting of all points which are either on the circle or lie inside the circle is called the circular region.

- A chord of a circle divides it into two parts. Each part is called a segment.
- The part containing the minor arc is called the minor segment, and the part containing the major arc is called the major segment.

- A quadrilateral of which all the four vertices lie on a circle is called a cyclic quadrilateral. The four vertices A, B, C and D are said to be concyclic points.

- Equal chords of a circle (or of congruent circles) subtend equal angles at the centre.
- If two chords of a circle subtend equal angles at the centre, then the chords are equal.
- The line drawn through the centre of a circle to bisect a chord is perpendicular to the chord.
- The perpendicular from the centre of a circle to a chord bisects the chord.
- Equal chords of a circle are equidistant from the centre whereas the equidistant chords from the centre are equal.
- Chords corresponding to equal arcs are equal.
- Congruent arcs of a circle subtend equal angles at the centre.
- The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part.
- Angles in the same segment are equal, whereas the angle in a semicircle is a right angle.
- The sum of either pair of opposite angles of a cyclic quadrilateral is 180º.
- If the opposite angles of a quadrilateral are supplementary, then the quadrilateral is cyclic.
- If a line segment joining two points subtends equal angles at two other points lying on the same side of the line containing the line segment, then the four points are cyclic.
Theorems Related to Circles:
(1) Prove that If two arcs of circle are congruent, then corresponding chords are equal.
Given: Arc PQ of a Circle C(O,r) and arc RS of another circle C(O′,r) such that PQ≅RS
To Prove:PQ = RS
Construction: Draw Line segment OP, OQ, O′R and O′S.
Proof:
Case-I When arc(PQ) and arc(RS) are minor Arcs
In triangle OPQ and O′RS, We have
OP = OQ = O′R = O′S = r [Equal radii of two circles]
∠POQ=∠RO′S arc(PQ)≅arc(RS)⇒m(arc(PQ))≅m(arc(RS))⇒∠POQ=∠RO′S
So by SAS Criterion of congruence, we have
ΔPOQ≅ΔRO′S
⇒PQ=RS
Case-II When arc(PQ) and arc(RS) are major arcs.
If arc(PQ), arc(RS) are major arcs, then arc(QP) and arc(SR) are Minor arcs.
So arc(PQ)≅arc(RS)
⇒ arc(QP)≅arc(SR)
⇒QP=SR
⇒PQ=RS
Hence, PQ≅RS⇒PQ=RS
(2) Prove that If two chords of a circle are equal, then their corresponding arcs are congruent.
Given: Equal chords, PQ of a circle C(O,r) and RS of congruent circle C(O′,r)
To Prove:arc(PQ)≅arc(RS), where both arc(PQ) and arc(RS) are minor, major or semi-circular arcs.
Construction: If PQ,RS are not diameters, draw line segments OP, OQ, O′R and O′S.
Proof:
Case I: when arc(PQ) and arc(RS) are diameters
In this case, PQ and RS are semi circle of equal radii, hence they are congruent.
Case II: When arc(PQ) and arc(RS) are Minor arcs.
In triangles POQ and RO′S, we have
PQ=RS
OP=O′R=r and OQ=O′S=r
So by SSS-criterion of congruence, we have
ΔPOQ≅ΔRO′S
⇒ ∠POQ=∠RO′S
⇒ m(arc(PQ))=m(arc(RS))
⇒ arc(PQ)≅arc(RS)
Case III: When arc(PQ) and arc(RS) are major arcs
In this case, arc(QP) And arc(SR)will be minor arcs.
PQ=RS
⇒ QP=SR
⇒ m(arc(QP))=m(arc(SR))
⇒ 360∘−m(arc(PQ))−360∘−m(arc(RS))
⇒ m(arc(PQ))−m(arc(RS))
⇒ arc(PQ)≅arc(RS)
Hence, in all the three cases, we have arc(PQ)≅arc(RS)
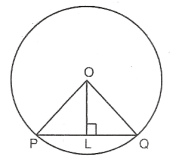
(3) Prove that The perpendicular from the centre of a circle to a chord bisects the chord.
Given: A Chord PQ of a circle C(O,r) and perpendicular OL to the chord PQ.
To Prove:LP=LQ
Construction: Join OP and OQ
Proof: In Triangles PLO and QLO, we have
OP=OQ=r [Radii of the same circle]
OL=OL [Common]
And, ∠OLP=∠OLQ [Each equal to 90∘]
So, by RHS-criterion of congruence, we have
ΔPLO≅ΔQLO
⇒ PL=LQ
(4) Prove that The line segment joining the centre of a circle to the mid-point of a chord is perpendicular to the chord.
Given: A Chord PQ OF a circle C(O,r) with mid-point M.
To Prove:OM⊥PQ
Construction: Join OP and OQ
Proof: In triangles OPM and OQM, we have
OP=OQ [Radii of the same circle]
PM=MQ [M is mid-point of PQ]
OM=OM
So, by SSC- criterion of congruence, we have
ΔOPM≅ΔOQM
⇒ ∠OMP=∠OMQ
But , ∠OMP+∠OMQ=180∘ [Linear pair]
⇒ ∠OMP+∠OMP=180∘ [∠OMP=∠OMQ]
⇒ 2∠OMP=180∘
⇒ ∠OMP=90∘
(5) Prove that There is one and only circle passing through three given points.
Given: Three non-collinear points P,Q and R.
To Prove: There is one and only one circle passing through P,Q and R.
Construction: Join PQ and QR. Draw perpendicular bisectors AL and BM of PQ and RQ respectively. Since P,Q and R. are not collinear. Therefore, the perpendicular bisectors AL and BM are not parallel.
Let AL And BM intersect at O. Join OP,OQ and OR.
Proof: Since O lies on the perpendicular bisector of PQ.
Therefore,
OP=OQ
Again, O Lies on the perpendicular bisector of QR.
Therefore,
OQ=OR
Thus, OP=OQ=OR=r (say)
Taking O as the centre draw a circle of radius s. Clearly, C(O,s) passes through P, Q and R. This proves that there is a circle passing the points P,Q and R.
We shall now prove that this is the only circle passing through P,Q and R.
If possible, let there be another circle with centre O′ and radius r, passing through the points P,Q and R. Then, O′ will lie on the perpendicular bisectors AL of PQ and BM of QR.
Since two lines cannot intersect at more than one point, so O′ must coincide with O. Since OP=r, O′P=s and O and O′ coincide, we must have
r=s
⇒ C(O,r)=C(O′,s)
Hence, there is one and only one circle passing through three non-collinear points P,Q and R.
(6) Prove that If two circles intersect in two points, then the through the centre is perpendicular to the common chord.
Given: Two circles C(O,r) and C(O′,s) intersecting at points A and B.
To Prove:OO′ is perpendicular bisector of AB.
Construction: Draw line segments
OA,OB,O′A and O′B
Proof: In triangles OAO′ and OBO′, we have
OA=OB=r
O′A=O′B=s
And, OO′=OO′
So, by SSS-criterion of congruence, we have
ΔOAO≅ΔOBO′
⇒ ∠AOO′=∠BOO′
⇒ ∠AOM=∠BOM [∠AOO′=∠AOM and ∠BOM=∠BOO′]
Let M be the point of intersection of AB and OO′
In triangles AOM and BOM, we have
OA=OB=r
⇒ ∠AOO′=∠BOO′
⇒ ∠AOM=∠BOM [∠AOO′=∠AOM and ∠BOM=∠BOO′]
Let M be the point of intersection of AB and OO′
In triangles AOM and BOM, we have
OA=OB=r
∠AOM=∠BOM
And OM=OM
So, by SAS-criterion of congruence, we have
ΔAOM≅ΔBOM
⇒ AM=BM and ∠AMO=∠BOM
But, ∠AOM+∠BMO=180∘
2∠AOM=180∘
⇒ ∠AOM=90∘
Thus, AM=BM and ∠AOM=∠BMO=90∘
Hence, OO′ is the perpendicular bisector of AB.
(7) Prove that Equal chords of a circle subtend equal angle at the centre.
Given: Two Chord AB and CD of circle C(O,r) such that AB=CDand OL⊥AB and OM⊥CD
To Prove: Chord AB and CD are equidistant from the centre O i.e OL=OM.
Construction: Join OA and OC.
Proof: Since the perpendicular from the centre of a circle to a chord bisects the chord.
Therefore,
OL⊥AB⇒AL=12AB..........(i)
And, OM⊥CD⇒CM=12CD..........(ii)
But, AB=CD
⇒ 12AB=12CD
⇒ AL=CM [Using (i) and (ii) ]….......(iii)
Now, in right triangles OAL and OCM, we have
OA=OC [Equal to radius of the circle]
AL=CM [From equation (iii)]
And, ∠ALO=∠CMO [Each equal to 90∘]
So by RHS criterion of convergence, we have
ΔOAL≅ΔOCM
⇒ OL=OM
Hence, equal chord of a circle are equidistant from the centre.
(8) Prove that Chords of a circle which are equidistant from the centre are equal.
Given: Two Chords AB and CD of a circle C(O,r) which are equidistant from its centre i.e. OL=OM, where OL⊥AB and OM⊥CD.
To Prove: Chords are Equal i.e. AB=CD
Construction: Join OA and OC
Proof: Since the perpendicular from the centre of a circle to a chord bisects the chord.
Therefore,
OL⊥AB
⇒ AL=BL
⇒ AL=12AB
And, OM⊥CD
⇒ CM=DM
⇒ CM=12CD
In triangles OAL and OCM, we have
OA=OC [Each equal to radius of the given Circle]
∠OLA=∠OMC [Each equal to 90∘]
And, OL=OM [Given]
So, by RHS, criterion of convergence, we have
ΔOAL≅ΔOCM
⇒ AL=CM
⇒ 12AL=12AB
⇒ AB=CD
Hence, the chords of a circle which are equidistant from the centre are equal.
(9) Prove that Equal chords of a circle subtend equal angle at the centre.
Given: A circle C(O,r) and its two equal chords AB and CD.
To Prove:∠AOB=∠COD
Proof:In triangles AOB and COD, we have
AB=CD [Given]
OA=OC [Each equal to r]
OB=OD [Each equal to r]
So, by SSC-criterion of Congruence, we have
ΔAOB≅ΔCOD
⇒ ∠AOB=∠COD
(15) Prove that If the angles subtended by two chords of a circle at the centre are equal, the chords are equal.
Given: Two Chord AB and CD of a circle C(O,r) such that ∠AOB=∠COD
To Prove: AB=CD
Proof: In triangles AOB and COD, we have
OA=OC [Each equal to r]
∠AOB=∠COD [Given]
OB=OD [Each equal to r]
So, by SAS-criterion of congruence, we have
ΔAOB≅ΔCOD
⇒ AB=CD
(11) Prove that Of any two chords of a circle, the larger chord is nearer to the centre.
Given: Two Chord AB and CD of a circle with Centre O such that AB>CD
To Prove: Chord AB is nearer to the centre of the circle i.e. OL<OM, where OL and OM are perpendiculars from O to AB and CD respectively
Construction: Join OA and OC.
Proof: Since the perpendicular from the centre of a circle to a chord bisects the chord. Therefore,
OL⊥AB⇒AL=12AB
And, OM⊥CD⇒CM=12CD
In right triangles OAL and OCM, we have
OA2=OL2+AL2
And, OC2=OM2+CM2
⇒ OL2+AL2=OM2+CM2.. (i) [OA=OC⇒OA2=OC2]
Now, AB>CD
⇒ 12AB>12CD
⇒ AL>CM
⇒ AL2>CM2
⇒ OL2+AL2>OL2+CM2 [Adding OL2 on both sides]
⇒ OM2+CM2>OL2+CM2 [using equation (i)]
⇒ OM2>OL2
⇒ OM>OL
⇒ OL<OM
Hence, AB is nearer to the centre than CD.
(12) Prove that Of any two chords of a circle, the chord nearer to the centre is larger.
Given: Two Chord AB and CD of a circle C(O,r) such that OL<OM, where OL and OM are perpendiculars From O on AB and CD respectively.
To Prove:AB>CD
Construction: Join OA and OC.
Proof: Since the perpendicular From the Centre of a circle to a chord bisects the chord.
AL=12AB and CM=12CD
In right triangles OAL and OCM, we have
OA2=OL2+AL2 and, OC2=OM2+CM2
⇒ AL2=OA2−OL2....... (i)
And, CM2=OC2−OM2.......(ii)
Now, OL<OM
⇒ OL2<OM2
⇒ −OL2>−OM2
⇒ OA2−OL2>OA2−OM2 [adding OA2 on both sides]
⇒ OA2−OL2>OC2−OM2 [OA2=OC2]
⇒ AL2>CM2
⇒ AL>CM
⇒ 2AL>2CM
⇒ AB>CD
(13) Prove that The angle subtended by an arc of a circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.
Given: An arc PQ of a circle C(O,r) and a point R on the remaining part of the circle i.e. arc QP.
To Prove:∠POQ=2∠PRQ
Construction: join RO and produce it to a point M outside the circle.
Proof: We shall consider the following three different cases:
Case I: when arc(PQ) is a minor arc.
We know that an exterior angle of a triangle is equal to the sum of the interior oppsite angles.
In ΔPOQ, ∠POM is the exterior angle.
∠POM=∠OPR+∠ORP
⇒∠POM=∠ORP+∠ORP [OP=OR=r, ∠OPR=∠ORP]⇒∠POM=2∠ORP.....................(i)
In ΔQOR, ∠QOM is the exterior angle.
∠QOM=∠OQR+∠ORQ
⇒∠QOM=∠OQP+∠ORQ [OQ=OR=r, ∠ORQ=∠OQR]
⇒∠QOM=2∠ORQ................(ii)
Adding equation (i) and (ii), we get
∠POM+∠QOM=2∠ORP+2∠ORP
⇒∠POM+∠QOM=2(∠ORP+∠ORP)
⇒ ∠POM=2∠PRQ
Case II: when arc(PQ) is a semi-circle
We know that an exterior angle of a triangle is equal to the sum of the interior opposite angles.
In ΔPOQ, we have
∠POM=∠OPR+∠ORP
⇒∠POM=∠ORP+∠ORP [OP=OR=r, ∠OPR=∠ORP]
⇒∠POM=2∠ORP.....................(iii)
In ΔQOR, We have
∠QOM=∠ORQ+∠OQR
⇒∠QOM=∠ORQ+∠ORQ [OQ=OR=r, ∠ORQ=∠OQR]
⇒∠QOM=2∠ORQ................(iv)
Adding equations (iii) and (iv), we get
∠POM+∠QOM=2(∠ORP+∠ORQ)
∠POQ=2∠PRQ
Case III: When arc(PQ) is a major arc.
We know that an exterior angle of a triangle is equal to the sum of the interior opposite angles
In ΔPOR, we have
∠POM=∠ORP+∠ORP [OP=OR=r, ∠OPR=∠ORP]
⇒∠POM=2∠ORP ...................(v)
In ΔQOR, we have
∠QOM=∠ORQ+∠OQR
⇒∠QOM=2∠ORQ ...................(vi)
Adding equations (v) and (vi), we get
∠POM+∠QOM=2(∠ORP+∠ORP)
⇒ Reflex ∠POQ=2∠PRQ
(14)Prove that Angles in the same segment of a circle are equal.
Given: A circle C(O,r), an arc PQ and two angles ∠PRQ and ∠PSQ in the same segment of the circle.
To Prove:∠PRQ= ∠PSQ
Construction: Join OP and OQ
Proof: we know that the angle subtended by an arc at the centre is double the angle subtended by the arc at any point in the remaining part of the circle. So we have
∠POQ=2∠PRQ and ∠POQ=2∠PSQ
⇒2∠PRQ=2∠PSQ
⇒∠PRQ=∠PSQ
We have
Reflex ∠POQ=2∠PRQ and ∠POQ=2∠PSQ
⇒2∠PRQ=2∠PSQ
⇒∠PRQ=∠PSQ
Thus , in both the cases, we have
∠PRQ=∠PSQ
(15) Prove that The angle in a semi-circle is a right angle.
Given:PQ is a diameter of a circle C(O,r) and ∠PRQ is an angle in semi-circle.
To Prove:∠POQ=90∘
Proof: we know that the angle subtended by an arc of a circle at its centre is twice the angle formed by the same arc at a point on the circle. So, we have
∠POQ=∠PRQ
⇒180∘=2∠PRQ [POQ is a straight line]
⇒∠PRQ=90∘
(16) Prove that The opposite angles of a cyclic quadrilateral are supplementary.
Given: A Cyclic quadrilateral ABCD
To Prove:∠A+∠C=180∘ and ∠B+∠D=180∘
Construction: Join AC and BD.
Proof: Consider side AB of quadrilateral ABCD as the Chord of the circle. Clearly, ∠ACB and ∠ADB are angles in the same segment determined by chord AB of the Circle.
∠ACB=∠ADB ………….(i)
Now , consider the side BC of quadrilateral ABCD as the chord of the circle. We find that ∠BAC and ∠BDC are angles in the same segment
∠BAC = ∠BDC [angles in the same segment are equal]..(ii)
Adding equation (i) and (ii), we get
⇒ ∠ACB+∠BAC=∠ADB+∠BDC
⇒ ∠ACB+∠BAC=∠ADC
⇒ ∠ABC+∠ACB+∠BAC=∠ABC+∠ADC
⇒ 180∘=∠ABC+∠ADC [sum of angle of triangle is 180∘ ]
⇒ ∠ABC+∠ADC=180∘
⇒ ∠B+∠D=180∘
But, ∠A+∠B+∠C+∠D=360∘
∠A+∠C=360∘−(∠B+∠D)
⇒∠A+∠C=360∘−180∘=180∘
Hence, ∠A+∠C=180∘ and ∠B+∠D=180∘
The converse of this theorem is also true as given below.
(17) Prove that If the sum of any pair of opposite angles of a quadrilateral is 180∘,then it is cyclic.
Given: A quadrilateral ABCD in which ∠B+∠D=180∘
To Prove: ABCD is acyclic quadrilateral.
Proof: If possible, Let ABCD be not cyclic quadrilateral. Draw a circle passing through three non-collinear points A, B and C. Suppose the circle meets AD or AD produced at D′. Join D′C.
Now, ABCD’ is a cyclic quadrilateral.
∠ABC+∠AD′C=180∘..............(i)
But, ∠B+∠D=180∘
i.e. ∠ABC+∠ADC=180∘..............(ii)
from (i) and (ii), we get
∠ABC+∠AD′C = ∠ABC+∠ADC
⇒ ∠AD′C = ∠ADC
⇒ An exterior angle of ΔCDD′ is equal to interior oppsite angle.
But, this is not possible, unless D′ coincides with D. Thus, the circle passing through A,B,C also passes through D.
Hence, ABCD is a cyclic Quadrilateral.
(18) Prove that If one side of a cyclic quadrilateral is produced, then the exterior angle is equal to the interior opposite angle.
Given: A Cyclic quadrilateral ABCD one of whose side AB is produced to E.
To Prove:∠CBE=∠ADC
Proof: Since ABCD is a quadrilateral and the sum of opposite pairs of angles in a cyclic quadrilateral is 180∘
∠ABC+∠ADC=180∘
But, ∠ABC+∠CBE=180∘ [Liner Pairs]
∠ABC+∠ADC=∠ABC+∠CBE
⇒ ∠ADC=∠CBE
Or, ∠CBE=∠ADC
(19) Prove that The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic.
Given: A Cyclic quadrilateral ABCD in which AP,BP,CR and DR are the bisectors of ∠A, ∠B, ∠C and ∠D respectively such that a quadrilateral PQRS is formed.
To Prove:PQRS is a cyclic quadrilateral.
Proof: In order to prove that PQRS is a cyclic quadrilateral, it is sufficient to show that
∠APB+∠CRD=180∘
Since the sum of the angles of a triangle is 180∘. Therefore, in triangles APB and CRD, we have
∠APB+∠PAB+∠PBA=180∘
And, ∠CRD+∠RCD+∠RDC=180∘
⇒ ∠APB+12∠A+12∠B=180∘
And, ∠CRD+12∠C+12∠D=180∘
⇒ ∠APB+12∠A+12∠B+∠CRD+12∠C+12∠D=180∘+180∘
∠APB+∠CRD+12{∠A+∠B+∠C+∠D}=360∘
∠APB+∠CRD+12{(∠A+∠C)+(∠B+∠D)}=360∘
∠APB+∠CRD+12(180∘+180∘)=360∘
∠APB+∠CRD=180∘
Hence, PQRS is a cyclic Quadrilateral.
(20) Prove that If two sides cyclic quadrilateral are parallel, then the remaining two sides are equal and the diagonals are also equal.
Given: A Cyclic quadrilateral ABCD in which AB∥DC.
To Prove: (i) AD=BC (ii) AC=BD
Proof: In order to prove the desired results, it is sufficient to show that ΔADC≅ΔBCD. Since ABCD is cyclic Quadrilateral and sum of opposite pairs of angles in a cyclic Quadrilateral is 180∘
∠B+∠D=180∘........(i)
Since AB∥DC and BC is a transversal and sum of the interior angles on the same side of a transversal is 180∘
∠ABC+∠BCD=180∘
∠B+∠C=180∘...................(ii)
From (i) and (ii), we get
∠B+∠D=∠B+∠C
⇒ ∠C=∠D.................(iii)
Now, consider triangles ADC and BCD. In ΔADC and ΔBCD, we have
∠ADC=∠BCD [From equation (iii)]
DC=DC [Common]
And, ∠DAC=∠CBD [∠DAC and ∠CBD are angles in the segment of chord CD]
So, by AAS-criterion of congruence, we have
ΔADC≅ΔBCD
⇒ AD=BC and AC=BD
(21) Prove that If two opposite sides of a cyclic quadrilateral are equal, then the other two sides are parallel.
Given: A cyclic quadrilateral ABCD such that AD=BC.
To Prove: AB∥CD
Construction: Join BD.
Proof: We have,
AD=BC
⇒ DA⌢≅BC⌢
⇒ m(DA)⌢≅(BC)⌢
⇒ 2∠2=2∠1
⇒ ∠2=∠1
But, these are alternate interior angles. Therefore, AB∥CD.
(22) Prove that An isosceles trapezium is cyclic.
Given: A trapezium ABCD in which AB∥DC and AD=BC
To Prove:ABCD is a cyclic trapezium.
Construction: Draw DE⊥AB and CF⊥AB.
Proof: In order to prove that ABCD is a cyclic trapezium, it is sufficient to show that ∠B+∠D=180∘.
In triangles DEA and CFB, we have
AD=BC [Given]
∠DEA=∠CFB [Each equal to 90∘]
And, DE=CF
So, by RHS-criterion of congruence, we have
ΔDEA≅ΔCFB
⇒ ∠A=∠B and ∠ADE=∠BCF
Now, ∠ADE=∠BCF
⇒ 90∘+∠ADE=90∘+∠BCF
⇒ ∠EDC+∠ADE=∠FCD+∠BCF
⇒ ∠ADC=∠BCD
⇒ ∠D=∠C
Thus, ∠A=∠B and ∠C=∠D.
∠A+∠B+∠C+∠D=360∘
⇒ 2∠B+2∠D=360∘
⇒ ∠B+∠D=180∘
Hence, ABCD is a cyclic quadrilateral.
...